Bianca Violet - Surfer Gallery
These images were created using SURFER. I first started playing around with this wonderful tool when I read about it in ‘Spektrum der Wissenschft’ and took part in the Surfer competition 2008.
Fórmula
- 0=x^{3}yz+x^{2}z^{2}y+5y^{3}z+5yx
Honey Bee
SURFER image properties:
brightness = 0, glow = 100
This picture won the SURFR competition in Kassel, hosted by ‘Spektrum der Wissenschaft’ und ‘Kasseler Sparkasse’ within the category ‘Users outside of Kassel’.

Fórmula
- ((x-3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y+3.5c) z) \cdot ((x+3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y-3.5c) z) \cdot ((x+3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y+3.5c)z) \cdot ((x-3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y-3.5c) z)
- (x^2 (y+3.5c)^2 + (y+3.5c)^2 (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y+3.5c) \cdot (z+3.5c)) \cdot (x^2 (y+3.5c)^2 + (y+3.5c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y+3.5c) \cdot (z-3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5c)^2 \cdot (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y-3.5c) \cdot (z+3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5*c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y-3.5c) \cdot (z-3.5c))
- ((x-3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x-3.5c)^2 \cdot (z+3.5c)^2 + (x-3.5c) y (z+3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x+3.5c)^2 \cdot (z-3.5c)^2 + (x+3.5c) y (z-3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x+3.5c)^2 \cdot (z+3.5c)^2 + (x+3.5c) y (z+3.5c)) \cdot ((x-3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x-3.5c)^2 \cdot (z-3.5c)^2 + (x-3.5c) y (z-3.5c))
- (x^2 y^2 + y^2 (z+5c)^2 + x^2 (z+5c)^2 + xy (z+5c)) \cdot (x^2 y^2 + y^2 (z-5c)^2 + x^2 (z-5c)^2 + xy (z-5c))
- (x^2 (y+5c)^2 + (y+5c)^2 z^2+ x^2 z^2 + x (y+5c) z) \cdot (x^2 (y-5c)^2 + (y-5c)^2 z^2 + x^2 z^2 + x (y-5c) z)
- ((x+5c)^2 y^2 + y^2 z^2 + (x+5c)^2 z^2 + (x+5c) yz) \cdot ((x-5c)^2 y^2 + y^2 z^2 + (x-5c)^2 z^2 + (x-5c) yz)
- c = 0.11
Clinch
The formula: x² y² + y² z² + x² z² + xyz = 0
describes a shape, which was discovered by Jakob Steiner. While he stayed in Rome in 1844, he studied its geometrical properties - hence it is called Roman surface. However, it was his friend Karl Weierstraß, who first published a paper on the surface and Steiner’s results in 1863, the year of Steiner’s death.
In the picture there are 18 Roman surfaces positioned symmetrically around the origin, each surface only slightly translated off the origin along one or two of the coordinate axes, so they intersect each other. Additionally, only a small portion of the whole arrangement is shown in this picture: everything within a small spherical neighborhood of the origin. Everything outside is simply cut off.
There are four blue ones, four red ones and four yellow ones, each color within one of the three coordinate planes. Their centers lie on the diagonal lines x= +/- y, y = +/- z, and z = +/- x respectively. The centers of the two orange, two green and two purple ones lie on the vertices of a cube, each color on one diagonal of the cube, matching the three coordinate axes.
Even thogh the Roman surface is non-orientable, which means there is no outside or inside - just one side, the SURFER program assigns two colors to it. Here the first color of each surface is picked as described above, and all surfaces have the same second color, which is black. Black is also the background color.

Fórmula
- ((x-3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x-3.5c)^2 \cdot (z+3.5c)^2 + (x-3.5c) y (z+3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x+3.5c)^2 \cdot (z-3.5c)^2 + (x+3.5c) y (z-3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x+3.5c)^2 \cdot (z+3.5c)^2 + (x+3.5c) y (z+3.5c)) \cdot ((x-3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x-3.5c)^2 \cdot (z-3.5c)^2 + (x-3.5c) y (z-3.5c))
- (x^2 (y+3.5c)^2 + (y+3.5c)^2 (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y+3.5c) \cdot (z+3.5c)) \cdot (x^2 (y+3.5c)^2 + (y+3.5c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y+3.5c) \cdot (z-3.5c))
- (x^2 (y-3.5c)^2 + (y-3.5c)^2 \cdot (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y-3.5c) \cdot (z+3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5*c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y-3.5c) \cdot (z-3.5c))
- ((x+5c)^2 y^2 + y^2 z^2 + (x+5c)^2 z^2 + (x+5c) yz) \cdot ((x-5c)^2 y^2 + y^2 z^2 + (x-5c)^2 z^2 + (x-5c) yz)
- (x^2 (y+5c)^2 + (y+5c)^2 z^2+ x^2 z^2 + x (y+5c) z) \cdot (x^2 (y-5c)^2 + (y-5c)^2 z^2 + x^2 z^2 + x (y-5c) z)
- (x^2 y^2 + y^2 (z+5c)^2 + x^2 (z+5c)^2 + xy (z+5c)) \cdot (x^2 y^2 + y^2 (z-5c)^2 + x^2 (z-5c)^2 + xy (z-5c))
- ((x+3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y-3.5c) z) \cdot ((x+3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x+3.5c)^2 z^2 + (x+3.5c)
Diamond

Fórmula
- 0=(x^{2}+y^{2}+z^{2}-5) \cdot (x^{2}+y^{2}+z^{2}-10) \cdot (x^{2}+y^{2}+z^{2}-15) \cdot (x^{2}+y^{2}+z^{2}-20) \cdot (x^{2}+y^{2}+z^{2}-25) \cdot (x^{2}-y^{2}+z^{2}- (a-0.5)) \cdot (xyz)^{2} \cdot (x+y) \cdot (x+z) \cdot (y+z) \cdot (x-y) \cdot (x-z) \cdot (y-z) -1000
- 0=(x^{2}+y^{2}+z^{2}-5) \cdot (x^{2}+y^{2}+z^{2}-10) \cdot (x^{2}+y^{2}+z^{2}-15) \cdot (x^{2}+y^{2}+z^{2}-20) \cdot (x^{2}+y^{2}+z^{2}-25) \cdot (x^{2}+y^{2}-z^{2}- (a-0.5)) \cdot (xyz)^{2} \cdot (x+y) \cdot (x+z) \cdot (y+z) \cdot (x-y) \cdot (x-z) \cdot (y-z) -1000
- 0=(x^{2}+y^{2}+z^{2}-5) \cdot (x^{2}+y^{2}+z^{2}-10) \cdot (x^{2}+y^{2}+z^{2}-15) \cdot (x^{2}+y^{2}+z^{2}-20) \cdot (x^{2}+y^{2}+z^{2}-25) \cdot (-x^{2}+y^{2}+z^{2}- (a-0.5)) \cdot (xyz)^{2} \cdot (x+y) \cdot (x+z) \cdot (y+z) \cdot (x-y) \cdot (x-z) \cdot (y-z) -1000
Mirror Ball
SURFER image properties:
a = 0.47, three surfaces colored red, yellow and blue, transparency = 60%, reflection = 100, various colorful lights :-)

Triple Winglet
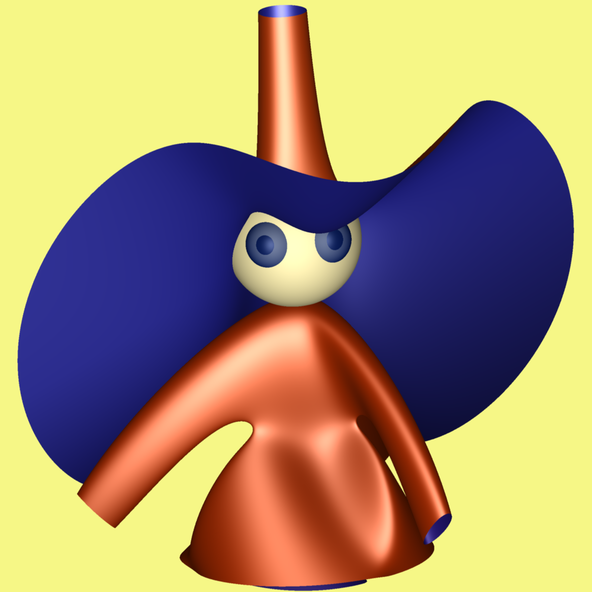
Fórmula
- 0=x^{3}-y^{3}-x^{2}z-y^{2}z-0.01
- 0=64(1-z)^3z^3-48(1-z)^2z^2(3x^2+3y^2+2z^2) +12(1-z)z(27(x^2+y^2)^2-24z^2 (x^2+y^2) +36 \cdot 1.4142yz(y^2-3x^2)+4z^4) + (9x^2+9y^2-2z^2) \cdot (-81 (x^2+y^2)^2-72z^2 (x^2+y^2) +108 \cdot 1.4142xz (x^2-3y^2))
- 0=x^2+y^2+ (z+0.23)^2 -0.07
- 0=((x+b)^2 +y^2+ (z+0.26)^2 -0.01) \cdot ((x+a)^2 + (y+c)^2 + (z+0.26)^2-0.01)
- 0=((x+b)^2 +y^2+ (z+0.26)^2 -0.005) \cdot ((x+a)^2+ (y+c)^2 + (z+0.26) ^2-0.005)
- a=0.09 \,\, b=0.2 \,\, c=-0.18 \,\,
Fairy

Fórmula
- x^2+y^2+z^2-3b
- ( (x+c)^2 \cdot (y-c)^2 + (x+c)^2 \cdot (z+c)^2 + (y-c) ^2 \cdot (z+c)^2 -3a (x+c) \cdot (y-c) \cdot (z+c)) \cdot ( (x-c) ^2 \cdot (y+c)^2 + (x-c) ^2 \cdot (z-c)^2 + (y+c)^2 \cdot (z-c)^2 -3a (x-c) \cdot (y+c) \cdot (z-c))
- ((x+c)^2 \cdot (y+c)^2 + (x+c)^2 \cdot (z-c)^2 + (y+c)^2 \cdot (z-c)^2 + 3a(x+c) \cdot (y+c) \cdot (z-c)) \cdot ((x-c)^2 \cdot (y-c)^2 + (x-c)^2 \cdot (z+c)^2 + (y-c)^2 \cdot (z+c)^2 +3a(x-c) \cdot (y-c) \cdot (z+c))
- ((x-c) ^2 \cdot (y+c)^2 + (x-c)^2 \cdot (z+c)^2 + (y+c)^2 \cdot (z+c)^2 -3a(x-c) \cdot (y+c) \cdot (z+c)) \cdot ((x+c)^2 \cdot (y-c)^2 + (x+c)^2 \cdot (z-c)^2 + (y-c)^2 \cdot (z-c)^2 -3a(x+c) \cdot (y-c) \cdot (z-c))
- ((x+c)^2 \cdot (y+c)^2 + (x+c)^2 \cdot (z-c)^2 + (y+c)^2 \cdot (z-c)^2 -3a(x+c) \cdot (y+c) \cdot (z-c)) \cdot ( (x-c)^2 \cdot (y-c)^2 + (x-c)^2 \cdot (z+c)^2 + (y-c)^2 \cdot (z+c)^2 -3a(x-c) \cdot (y-c) \cdot (z+c))
- ((x-c)^2 \cdot (y+c)^2 + (x-c)^2) \cdot (z+c)^2 + (y+c)^2 \cdot (z+c)^2 +3a(x-c) \cdot (y+c) \cdot (z+c)) \cdot ((x+c)^2 \cdot (y-c)^2 + (x+c)^2 \cdot (z-c)^2 + (y-c)^2 \cdot (z-c)^2 +3a(x+c) \cdot (y-c) \cdot (z-c)) \cdot ( (x+c)^2 \cdot (y-c)^2 + (x+c)^2 \cdot (z+c)^2 + (y-c)^2 \cdot (z+c)^2 +3a(x+c) \cdot (y-c) \cdot (z+c)) \cdot ((x-c)^2 \cdot (y+c)^2 + (x-c)^2 \cdot (z-c)^2 + (y+c)^2 \cdot (z-c)^2 +3a(x-c) \cdot (y+c) \cdot (z-c))
- a=0.65; \,\, b=0.45; \,\, c=0.9
Flower

Fórmula
- ((x-3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y+3.5c) z) \cdot ((x+3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y-3.5c) z) \cdot ((x+3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y+3.5c)z) \cdot ((x-3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y-3.5c) z)
- (x^2 (y+3.5c)^2 + (y+3.5c)^2 (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y+3.5c) \cdot (z+3.5c)) \cdot (x^2 (y+3.5c)^2 + (y+3.5c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y+3.5c) \cdot (z-3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5c)^2 \cdot (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y-3.5c) \cdot (z+3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5*c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y-3.5c) \cdot (z-3.5c))
- ((x-3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x-3.5c)^2 \cdot (z+3.5c)^2 + (x-3.5c) y (z+3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x+3.5c)^2 \cdot (z-3.5c)^2 + (x+3.5c) y (z-3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x+3.5c)^2 \cdot (z+3.5c)^2 + (x+3.5c) y (z+3.5c)) \cdot ((x-3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x-3.5c)^2 \cdot (z-3.5c)^2 + (x-3.5c) y (z-3.5c))
- (x^2 y^2 + y^2 (z+5c)^2 + x^2 (z+5c)^2 + xy (z+5c)) \cdot (x^2 y^2 + y^2 (z-5c)^2 + x^2 (z-5c)^2 + xy (z-5c))
- (x^2 (y+5c)^2 + (y+5c)^2 z^2+ x^2 z^2 + x (y+5c) z) \cdot (x^2 (y-5c)^2 + (y-5c)^2 z^2 + x^2 z^2 + x (y-5c) z)
- ((x+5c)^2 y^2 + y^2 z^2 + (x+5c)^2 z^2 + (x+5c) yz) \cdot ((x-5c)^2 y^2 + y^2 z^2 + (x-5c)^2 z^2 + (x-5c) yz)
- c = 0.16
Package

Blue Planet

Fórmula
- x^2+y^2+z^2-0.62
- ((x-3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y+3.5c) z) \cdot ((x+3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y-3.5c) z) \cdot ((x+3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y+3.5c)z) \cdot ((x-3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y-3.5c) z)
- (x^2 y^2 + y^2 (z+5c)^2 + x^2 (z+5c)^2 + xy (z+5c)) \cdot (x^2 y^2 + y^2 (z-5c)^2 + x^2 (z-5c)^2 + xy (z-5c))
- (x^2 (y+5c)^2 + (y+5c)^2 z^2+ x^2 z^2 + x (y+5c) z) \cdot (x^2 (y-5c)^2 + (y-5c)^2 z^2 + x^2 z^2 + x (y-5c) z)
- ((x+5c)^2 y^2 + y^2 z^2 + (x+5c)^2 z^2 + (x+5c) yz) \cdot ((x-5c)^2 y^2 + y^2 z^2 + (x-5c)^2 z^2 + (x-5c) yz)
- (x^2 (y+3.5c)^2 + (y+3.5c)^2 (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y+3.5c) \cdot (z+3.5c)) \cdot (x^2 (y+3.5c)^2 + (y+3.5c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y+3.5c) \cdot (z-3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5c)^2 \cdot (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y-3.5c) \cdot (z+3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5*c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y-3.5c) \cdot (z-3.5c))
- ((x-3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x-3.5c)^2 \cdot (z+3.5c)^2 + (x-3.5c) y (z+3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x+3.5c)^2 \cdot (z-3.5c)^2 + (x+3.5c) y (z-3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x+3.5c)^2 \cdot (z+3.5c)^2 + (x+3.5c) y (z+3.5c)) \cdot ((x-3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x-3.5c)^2 \cdot (z-3.5c)^2 + (x-3.5c) y (z-3.5c))
- c = 0.12
Red Planet
Roman Candy
The Real Projective Plane is the space of lines in real three-dimensional space (R3) passing through the origin. When the mathematician Jakob Steiner stayed in Rome, he thought of a mapping of the Real Projective Plane into R3. The resulting surface intersects itself. It is now called Roman Surface or Steiner Surface.
There is a triple point in the origin and each of the three coordinate planes is tangential to the surface. Apart from the origin, the segments along the coordinate axes are double points, which terminate in six pinch points.
In the picture you see a yellow Roman Surface surrounded by six parts of the Roman Surface, meeting at the pinchpoints, emphasizing the high degree of symmetry of the surface.

Fórmula
- x^2+y^2+z^2-0.62
- ((x-3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y+3.5c) z) \cdot ((x+3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y-3.5c) z) \cdot ((x+3.5c)^2 \cdot (y+3.5c)^2 + (y+3.5c)^2 z^2+ (x+3.5c)^2 z^2 + (x+3.5c) \cdot (y+3.5c)z) \cdot ((x-3.5c)^2 \cdot (y-3.5c)^2 + (y-3.5c)^2 z^2 + (x-3.5c)^2 z^2 + (x-3.5c) \cdot (y-3.5c) z)
- (x^2 y^2 + y^2 (z+5c)^2 + x^2 (z+5c)^2 + xy (z+5c)) \cdot (x^2 y^2 + y^2 (z-5c)^2 + x^2 (z-5c)^2 + xy (z-5c))
- (x^2 (y+5c)^2 + (y+5c)^2 z^2+ x^2 z^2 + x (y+5c) z) \cdot (x^2 (y-5c)^2 + (y-5c)^2 z^2 + x^2 z^2 + x (y-5c) z)
- ((x+5c)^2 y^2 + y^2 z^2 + (x+5c)^2 z^2 + (x+5c) yz) \cdot ((x-5c)^2 y^2 + y^2 z^2 + (x-5c)^2 z^2 + (x-5c) yz)
- (x^2 (y+3.5c)^2 + (y+3.5c)^2 (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y+3.5c) \cdot (z+3.5c)) \cdot (x^2 (y+3.5c)^2 + (y+3.5c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y+3.5c) \cdot (z-3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5c)^2 \cdot (z+3.5c)^2 + x^2 (z+3.5c)^2 + x (y-3.5c) \cdot (z+3.5c)) \cdot (x^2 (y-3.5c)^2 + (y-3.5*c)^2 \cdot (z-3.5c)^2 + x^2 (z-3.5c)^2 + x (y-3.5c) \cdot (z-3.5c))
- ((x-3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x-3.5c)^2 \cdot (z+3.5c)^2 + (x-3.5c) y (z+3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x+3.5c)^2 \cdot (z-3.5c)^2 + (x+3.5c) y (z-3.5c)) \cdot ((x+3.5c)^2 y^2 + y^2 (z+3.5c)^2 + (x+3.5c)^2 \cdot (z+3.5c)^2 + (x+3.5c) y (z+3.5c)) \cdot ((x-3.5c)^2 y^2 + y^2 (z-3.5c)^2 + (x-3.5c)^2 \cdot (z-3.5c)^2 + (x-3.5c) y (z-3.5c))
- c=0.15
Wheel of Time
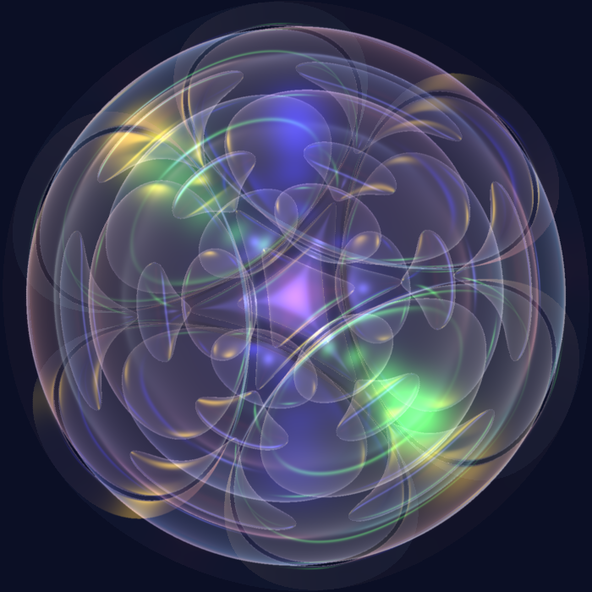
Fórmula
- 0=(x^{2}+y^{2}+z^{2}-5b) \cdot (x^{2}+y^{2}+z^{2}-10b) \cdot (x^{2}+y^{2}+z^{2}-15b) \cdot (x^{2}+y^{2}+z^{2}-20b) \cdot (x^{2}+y^{2}+z^{2}-25b) \cdot (x^{2}+y^{2}-z^{2} + 0.5)) \cdot (x^{2}-y^{2}+z^{2} + 0.5)) \cdot (-x^{2}+y^{2}+z^{2} + 0.5)) -100
Soap Bubble
SURFER image properties:
b = 0.38, transparency = 90%, colorful lights
Slice of Boy
Imagine sewing the edge of a Möbius strip, which is just one closed curve, to the boundary of a disk! The resulting closed surface still contains the Möbius strip and will therefore be non-orientable (you cannot tell what is the outside or the inside - it is all the same). That is why it must have selfintersections in our real three-dimensional space. But can it be done smoothly with no sharp or pointy edges?
The famous mathematician David Hilbert assigned his student Werner Boy to prove that it is impossible. To the surprise of his teacher, Boy constructed such a smooth surface in 1901. He described it by giving the corresponding curves of its intersection with a family of parallel planes.
Now in this picture, you see a slice of two Boy surfaces (one being the mirror image of the other), cut not by planes but by two spheres with the same center and of slightly different radii.
The equation used for this visualization of the Boy surface was found by François Apéry.
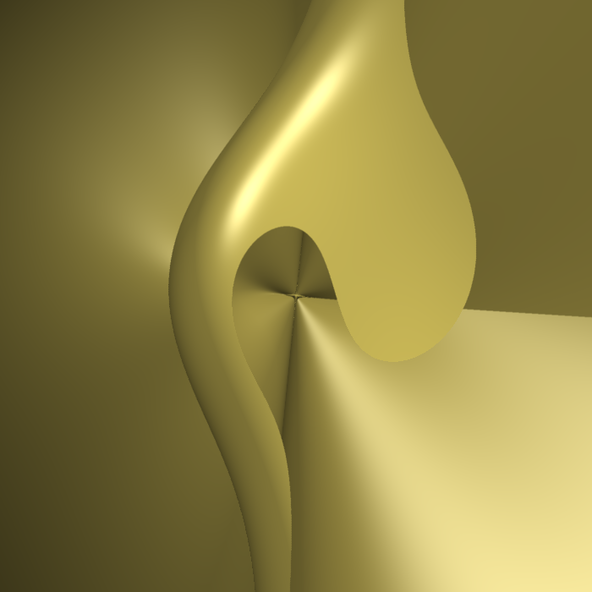
Fórmula
- 0=(-x^{3}-y^{2}z-z^{3}+x^{2}) \cdot (x^{3}+y^{2}z+z^{3}+x^{2})^{2}-x^{2}y^{2}z^{2}
Yin Yang

Fórmula
- 0=(((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} -2(y+c) -1)^{2}-8(z+5a) ^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} -2(y+c) -1))^{2}
- 0=(((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2}+2(y+c) -1) \cdot (((x+15b)^{2} + (y+c) ^{2} + (z+5a)^{2} - 2(y+c) -1)^{2}-8(z+5a) ^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b) ^{2} + (y+c)^{2} + (z+5a)^{2}-2(y+c) -1) +d)^{2} \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b) ^{2} + (y+c)^{2}+ (z+5a)^{2} -2(y+c) -1)^{2}-8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} -2(y+c) -1) -d)
- 0=(((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2}+2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2}+ (z+5a)^{2} - 2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a) ^{2} - 2(y+c) -1) +2d)^{2} \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a) ^{2} + 2(y+c) -1) \cdot (((x+15b) ^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1) -2d)
- 0=(((x+15b)^{2} + (y+c)^{2} + (z+5a) ^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} -2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2}+ (z+5a)^{2} - 2(y+c) -1) +3d)^{2} \cdot (((x+15b)^{2}+ (y+c)^{2} + (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a) ^{2} - 2(y+c) -1) -3d)
- 0=(((x+15b)^{2}+ (y+c)^{2}+ (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} -2(y+c) -1)^{2}-8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1) +4d)^{2} \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} -2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1) -4d)
- 0=(((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1) +5d)^{2} \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} +2(y+c) -1) \cdot (((x+15b)^{2} + (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1)^{2} - 8(z+5a)^{2}) +16(x+15b) \cdot (z+5a) \cdot ((x+15b)^{2}+ (y+c)^{2} + (z+5a)^{2} - 2(y+c) -1) -5d)
- a=b=0 \,\, c=0.47 \,\, d=2.5
Tunnel Vision