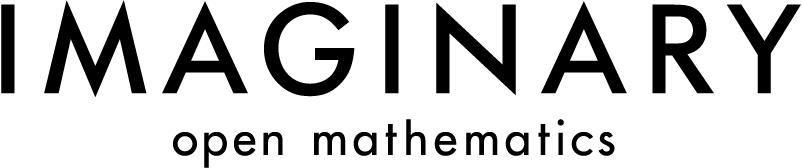The Italian premiere of a large IMAGINARY exhibition
Oliver Labs
Oliver Labs is a geometer specialized in algorithmical aspects. This includes the construction of interesting curves and surfaces, their visualization and the study of any appearing algorithmic problems. These pictures are algebraic surfaces with (amazingly) many singularities.

Formula
- P_6 − αK^2 = 0
- P_6 = ( τ^2x^2−y^2)( τ^2y^2−z^2)( τ^2z^2− x^2), τ = (1+\sqrt{5})/2
- α = (2τ+1)/4=(2+\sqrt{5})/4
- K = x^2+y^2+z^2−1
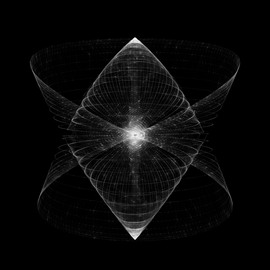
Barth Sextic
This surface of degree 6 (sextic) was constructed by Wolf Barth in 1996. Altogether, it has 65 singularities when also counting the 15 invisible ones which are infinitely far away. 65 is the maximum possible number of singularities on a sextic as shown in 1997 by Jaffe and Ruberman.
Barth’s construction was a big surprise: For a long time geometers believed that a surface of degree 6 cannot have more than 64 singularities. The icosahedral symmetry is one of the most striking features of Barth’s sextic. But not all sextics with 65 have this kind of symmetry; there even exists a 3–parameter family of surfaces with 65 singularities! In this family one can choose three parameters almost at random and always obtains a sextic with 65 singularities. The exact equation of Barth’s sextic is P6 − αK2 = 0, where P6 = ( τ2x2−y2)( τ2y2−z2)( τ2z2− x2), τ= (1+√5)/2 is the golden ratio, α = (2τ+1)/4=(2+√5)/4 and K = x2+y2+z2−1 describes a sphere of radius 1.
Formula
- x^7-21*x^5* y^2+35*x^3* y^4-7*x*y^6+7* x^6*1+21*x^4* y^2*1+21*x^2* y^4*1+7*y^6* 1-57*x^4*1^3-114* x^2*y^2*1^3-57* y^4*1^3+(24/7* a^2+768/49* a+800/7)*x^2* 1^5+(24/7*a^2+768/49* a+800/7)*y^2* 1^5+(-149808/2401* a^2+3216/343* a-147584/2401)* 1^7+(-49*a^2+7* a-52)*x^4*1^2* z+(-98*a^2+14* a-104)*x^2* y^2*1^2*z+(-49* a^2+7*a-52)* y^4*1^2*z+(128/7* a^2+704/49* a+128/7)*x^2* 1^4*z+(128/7* a^2+704/49* a+128/7)*y^2* 1^4*z+(-1632/343* a^2+16/7*a-192/343)* 1^6*z+(-98* a^2+14*a-101)* x^4*1*z^2+(-196* a^2+28*a-202)* x^2*y^2*1*z^2+(-98* a^2+14*a-101)* y^4*1*z^2+(3016/7* a^2-2904/49* a+440)*x^2* 1^3*z^2+(3016/7* a^2-2904/49* a+440)*y^2* 1^3*z^2+(-17440/343* a^2+416/49* a-17040/343)* 1^5*z^2+(-49* a^2+7*a-50)* x^4*z^3+(-98* a^2+14*a-100)* x^2*y^2*z^3+(-49* a^2+7*a-50)* y^4*z^3+(5776/7* a^2-5648/49* a+5888/7)*x^2* 1^2*z^3+(5776/7* a^2-5648/49* a+5888/7)*y^2* 1^2*z^3+(-313136/343* a^2+6288/49* a-319264/343)* 1^4*z^3+(3680/7* a^2-3608/49* a+536)*x^2* 1*z^4+(3680/7* a^2-3608/49* a+536)*y^2* 1*z^4+(-592240/343* a^2+11856/49* a-603856/343)* 1^3*z^4+(816/7* a^2-800/49* a+832/7)*x^2* z^5+(816/7* a^2-800/49* a+832/7)*y^2* z^5+(-458832/343* a^2+1312/7* a-467840/343)* 1^2*z^5+(-166272/343* a^2+3328/49* a-169536/343)* 1*z^6+(-166272/2401* a^2+3328/343* a-169536/2401)* z^7
Labs Septic
In 2004, while writing his Ph. D. thesis at Mainz, Oliver Labs constructed a surface of degree 7 (septic) with 99 singularities. Since 1997 one knows for surfaces of degree 6 that the maximum possible number of singularities is 65. In degree 7 there is a result of A. N. Varchenko from 1982 which tells us that there cannot be more than 104 singularities. In 1992, Chmutov constructed a septic with 93 singularities which was the world record at that time. Since Labs’ construction, this world record has been 99. It is open if septics can have 100, 101, … , 104 singularities.
The Labs Septic has the symmetry of a regular 7–gon. However, similar to Duco van Straten’s computation for the sextics, one can compute that there is indeed a 5–parameter family of septics with 99 singularities. For the construction of the septic Oliver Labs used the computer algebra software Singular which is developed at the technical university of Kaiserslautern. This software is very well suited for working with singular algebraic surfaces.

Formula
- S_5(x, y)+t(z) = 0\\ S_5(x, y) = x^5−10x^3y^2+5xy^4−5x^4−10x^2y^2− 5y^4+20x^2+20y^2−16\\ t(z) = −3z^5+10z^3−15z−8
Quintic with 15 cusps
This surface of degree 5 (quintic) has 15 singularities whose type is called ordinary cusp or A2. The surface is part of a series of related surfaces of infinitely many degrees constructed by Oliver Labs in 2005. As one can see from the picture, five of the singularities look different than the others. These five ones are more specifically of type A2++ and the others of type A2+−. The former can be described locally by the equation x3+y2+z2 = 0, the others by x3+y2−z2 = 0. The equation of the quintic with 15 cusps is S5(x, y)+t(z) = 0, where S5(x, y) = x5−10x3y2+5xy4−5x4−10x2y2− 5y4+20x2+20y2−16 is a regular pentagon, and where the polynomial t(z) = −3z5+10z3−15z−8 is a variant of the so–called Tchebychev Polynomials.
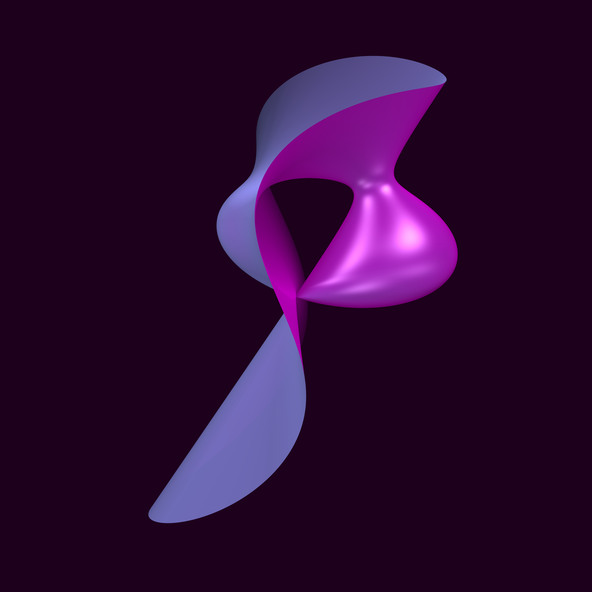
Togliatti Quintic
Eugenio Giuseppe Togliatti proved in 1937 that a surface of degree 5 (quintic) with exactly 31 singularities exists - a world record at that time.
In 1980 it was Arnaud Beauville who used an interesting relation to coding theory in order to show the non-existence of a quintic with more singularities. This means that Togliatti’s world record can never be improved!
The equation used here was found by Wolf Barth in 1990.
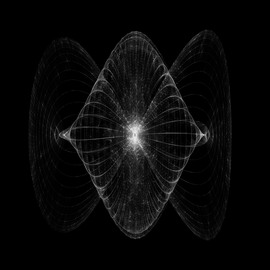
Kummer Quartic
In 1875, Eduard Kummer was the first person who explicitly asked the question of what the maximum number of singularities on a surface of a specific degree are. In his case the degree was 4 and are called quartics.
He showed that for quartics, there can not be more than 16 singularities.
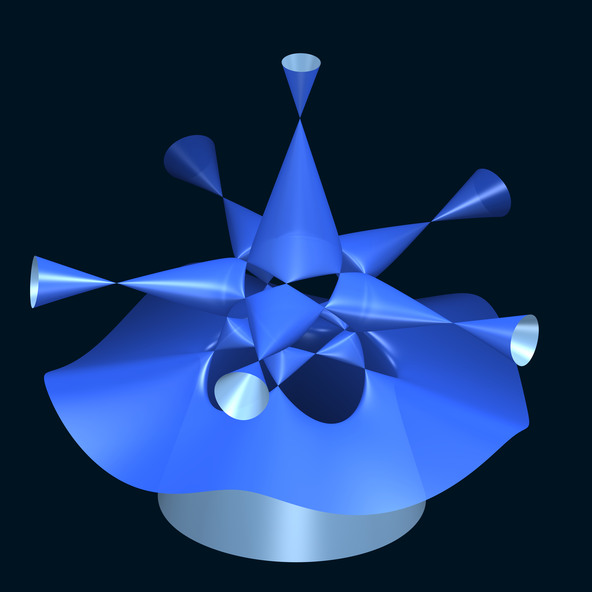
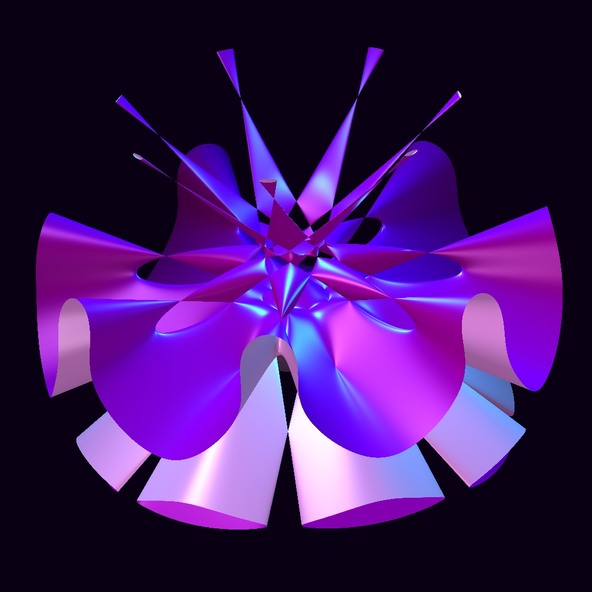
A cubic surface with a D4 singularity
Equation: classical (19th century), adapted by Oliver Labs.
Visualization: Oliver Labs