Ulrich Pinkall, Nicholas Schmitt, Charles Gunn and Tim Hoffmann
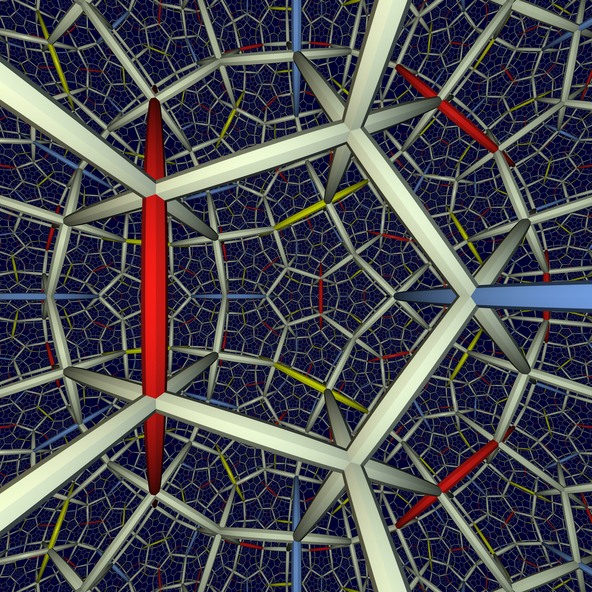
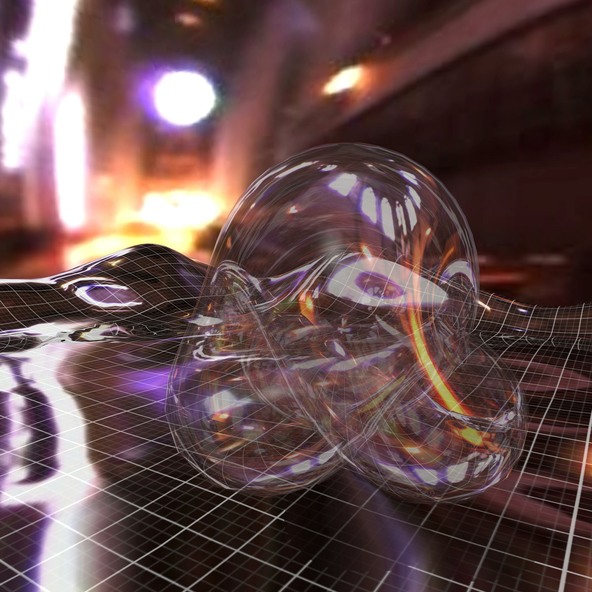
Differential geometry is the study of curved shapes, like curved lines or surfaces in space, or even shapes with many dimensions. From the viewpoint of differential geometry the shapes of soap films and soap bubbles are singled out by the fact that the so-called “mean curvature” is the same at every point of the surface. Several pictures in this gallery show surfaces of constant mean curvature, one picture shows a surface that does not have minimal area but minimal “total curvature”. Another picture shows a three dimensional space of constant curvature.
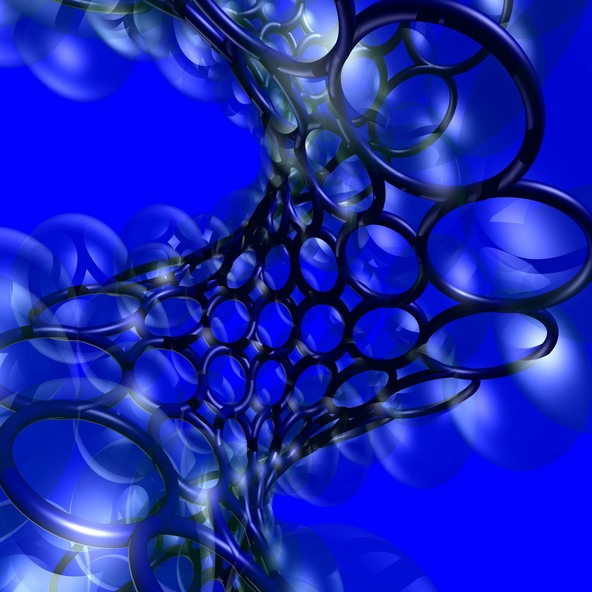
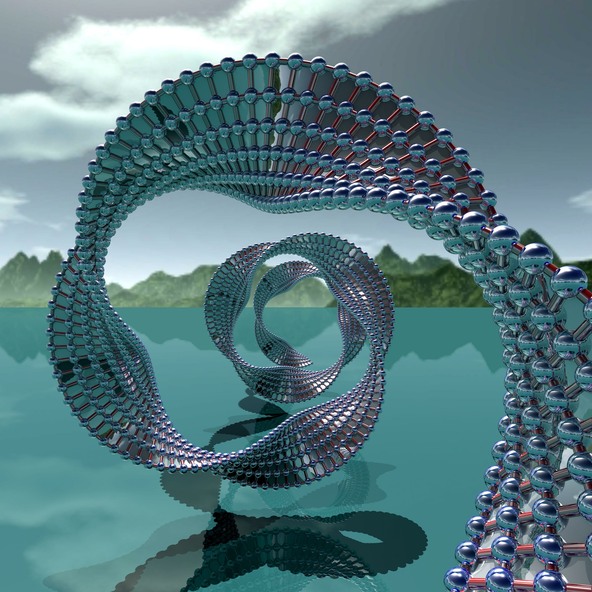
Discrete Minimal Surface
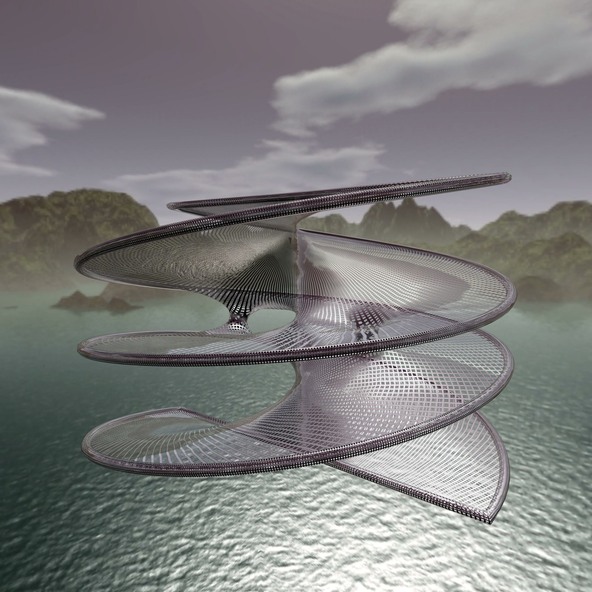
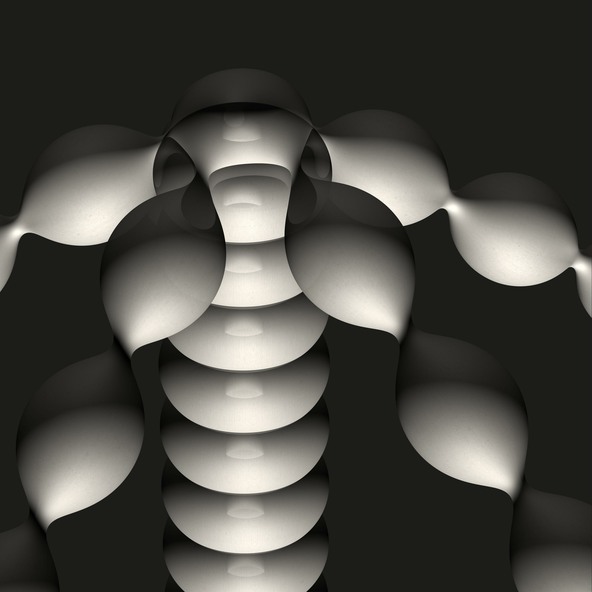
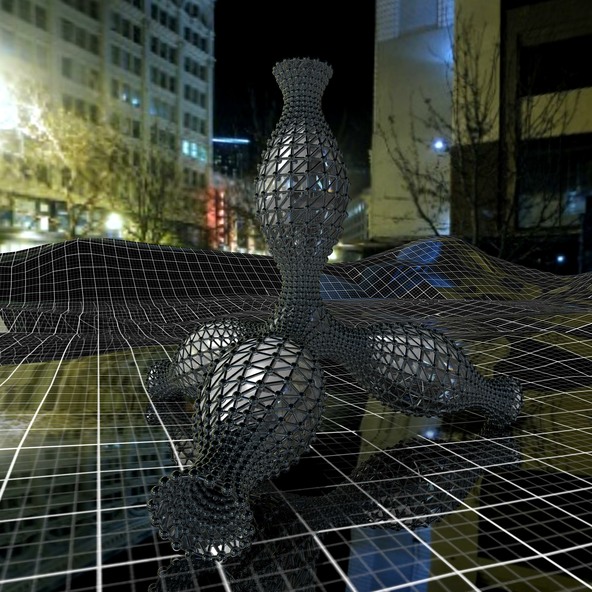
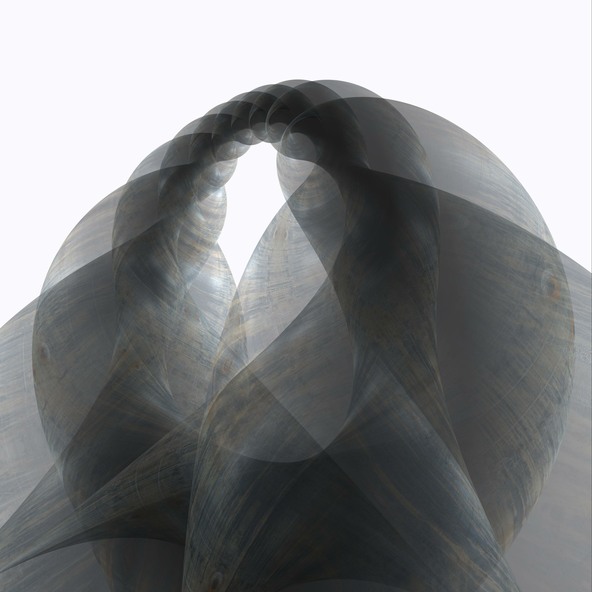
Minimal surfaces are a classical subject matter of differential geometry. They are surfaces whose mean curvature vanishes everywhere. The best known among this surface class are the Catenoid and the Helicoid. One of the many interesting features of minimal surfaces is the existence of an associated family. This means that the surfaces are deformed in such a way that they remain minimal (they are even isometrical to each other and corresponding tangent planes are parallel). Helicoid and Catenoid belong to the same associated family. As a result, they can be deformed into one another and in between surfaces are minimal, as well.
The image shows a discretization of the minimal surface half way between Catenoid and Helicoid. It is composed of spheres and circles touching each other at their points of contact. There is also an associated family for these discrete minimal surfaces and both the radii of corresponding spheres and the positions of corresponding circle disks are equal.
The scene was developed with jReality, the image itself was calculated by POV- Ray.