Diplotori - flat polyhedral tori
hands-on
Licence
Credits
- Design - Development - implementation - Programming
A 3d-printed antiprism diplotorus with 12 vertices
A paper-folded prism diplotorus with 14 vertices
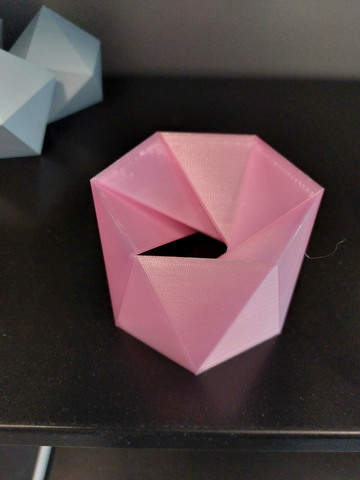
Diplotori are polyhedra “of genus one” (that is, “with one hole”, like a buoy or a donut) which are “flat” in the sense that the total angle at each vertex is exactly 360 degrees.
These tori are flat in the sense that the total angle at each vertex is 360 degrees.
We provide files to produce patterns that can fold into these tori in several ways.
One way is to print the pattern as an image, then cut with scissors or a paper knife and crease and fold by hand.
Another way is to send an image to an automatic paper cutter, which, by controlling a blade or a laser beam, can cut and pre-crease the paper very precisely.
We also provide shape files which can be used to 3d-print diplotori.
These flat tori, apparently introduced by Ulrich Brehm in 1978, were not thoroughly studied until around 2020.
They have since been discovered many times, and called “Genus one polyhedra whose Gaussian curvature is zero at all vertices”, “everywhere flat polyhedral tori in E3”, “toroidal isogonal polyhedra”, “basis-free isogonal prismatoids”, “acoptic isogonal prismatoids”, “polyhedral isometric embeddings of flat tori”, “origami tori”, “iris toroids”, and possibly other names, and we call them “diplotori”.
Any flat torus (abstractly obtained by gluing opposite sides of a parallelogram) can be realized as a diplotorus.
